Exploring Wolfram’s Theory of Everything: Could the Universe Be a Giant Computer Program?
For several years, I’ve been asked to explore Stephen Wolfram’s “Theory of Everything”—a bold attempt to describe the fundamental workings of the universe through computational theory. Wolfram, a renowned computer scientist and creator of the popular Mathematica software, proposes that the universe operates like a computer program. What if, at its core, the universe follows a set of simple computational rules that give rise to the complex phenomena we observe today such as gravity, the Standard Model, and relativity? This notion connects closely to the simulation hypothesis, which speculates that our reality is a giant simulation. It’s an intriguing theory, but does it hold up under scientific scrutiny?
The Simulation Hypothesis and Computation in Physics
The basic idea behind Wolfram’s theory is simple, yet profound: the universe follows a set of fundamental rules, not unlike a computer algorithm. From these basic rules, everything—from the force of gravity to the behavior of subatomic particles—emerges. Wolfram’s approach is based on similar principles that underpin cellular automaton, where simple rules can generate surprisingly complex patterns.
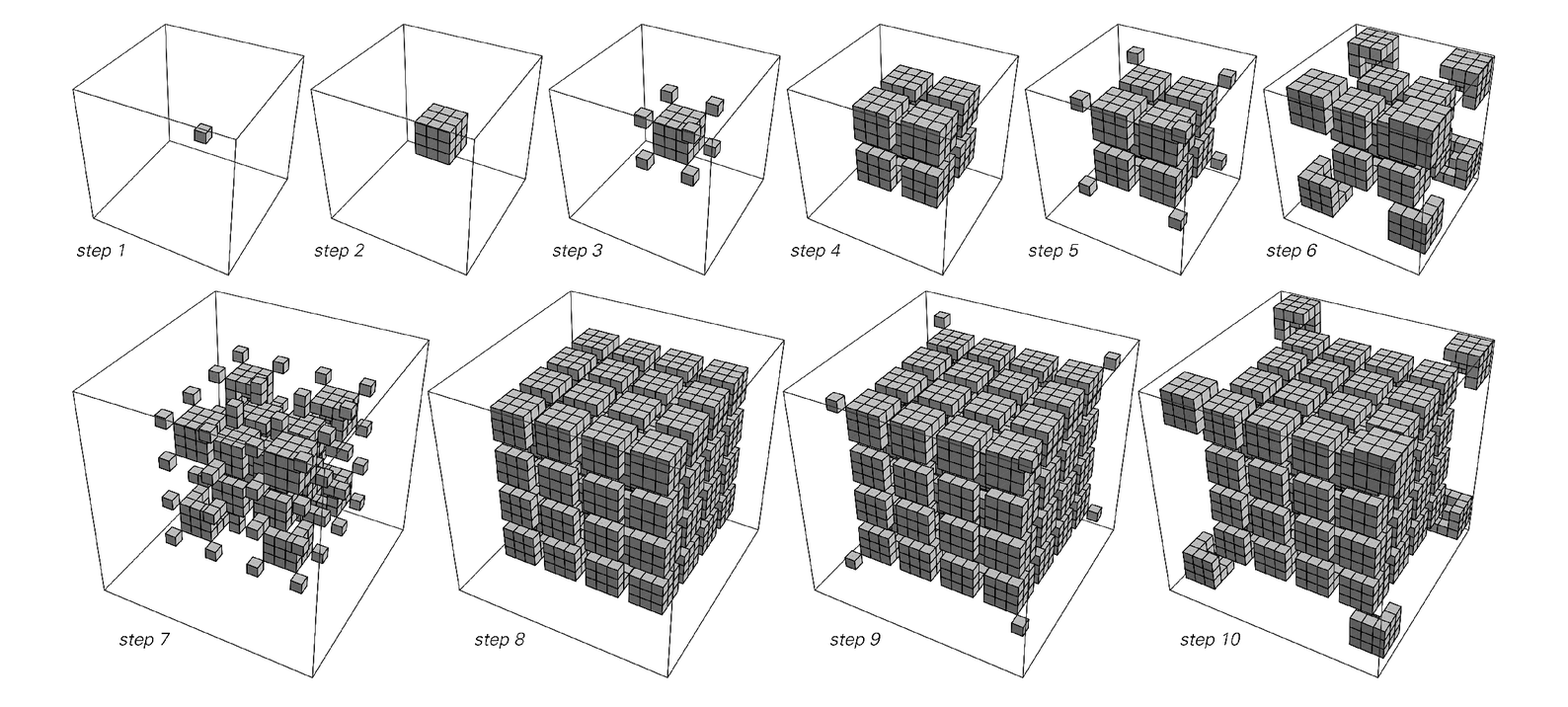
But can we actually explain physics with computation? Wolfram suggests that you could conceptualize the universe in a manner similar to a cellular automaton—essentially a four-dimensional “code” that evolves step by step. This would mean that all aspects of the universe are, at some level, deterministic and computationally structured. Yet, despite the elegance of this idea, it faces significant hurdles when subjected to the rigorous demands of physics, particularly Einstein’s theory of relativity.
Challenges with General Relativity and Lorentz Symmetry
The main challenge with Wolfram’s theory is its compatibility with Einstein’s General Relativity. Relativity describes how space and time are intimately connected and varies based on an observer’s reference frame. One major problem with computational approaches is that they work in discrete steps—increments of space and time. However, according to relativity, these increments can’t remain hidden. If space and time were truly discrete, this would manifest observationally. Yet, there’s no evidence of such discreteness.
In an elegant illustration, think of a photon—a quantum of light. The energy of the photon depends on how it’s observed: its energy is higher if the observer is moving towards it, lower if moving away. In an inherently discrete computational model, issues arise because different observers would calculate unobserved gaps in the graph representing space. This disconnect prevents any computational model from approximating General Relativity in a way that respects all of Einstein’s symmetries.
This brings us to the concept of Lorentz Symmetry, a key pillar of General Relativity that ensures the same physical laws apply regardless of how fast you’re moving or where you are in space. Attempting to simulate this through computational methods like grids, graphs, or even Wolfram’s hypergraphs has, thus far, proven problematic.
Introducing Hypergraphs: A Way Forward?
Despite the difficulties, Wolfram has pressed forward and introduced the idea of hypergraphs as a potential solution. A hypergraph is a more complex version of a traditional graph, where sets of nodes (representing points in space-time) are connected in ways that circumvent the discretization problems of simpler models. According to Wolfram, hypergraphs may offer a way to reconcile computation with both space and matter without breaking the theory of relativity.
In 2020, Wolfram and his collaborators published a follow-up to address concerns about Lorentz symmetry. They’ve focused on how hypergraphs might solve the problem of preserving the symmetry requirements of relativity in a discrete structure. In principle, this should work similarly to another theory in physics known as “causal sets,” a respected attempt to describe space and time as a network of discrete points with causal relationships between them.
At this juncture, it’s worth noting that while Wolfram’s hypergraphs offer a clever solution, they still leave much unexplained. For instance, his framework for quantum mechanics, the behavior of elementary particles, and how exactly the Standard Model fits into the picture remains vague. This is an area I hope to see more developments on, as successful integration here would truly validate or break his theory’s foundation.
Looking Ahead: Are We Near a Theory of Everything?
Wolfram’s theory is an ongoing, evolving effort, and it’s not without merit. At the very least, it introduces novel approaches to theoretical physics and stimulates some intriguing discussions. However, it hasn’t reached a point where it effectively competes with established theories like General Relativity or String Theory. Incorporating quantum physics and providing stringent mathematical proofs for his model remains a significant challenge. Time will tell whether Wolfram’s work is able to capture the attention of mainstream physicists or if it will remain a curious side-note in the long and complicated quest for a “Theory of Everything.”
In conclusion, Wolfram’s theory is an ambitious attempt to bring the simulation hypothesis into the realm of physics. Does it answer all the questions? Certainly not yet. But given the originality and clarity of thought, it’s certainly worth paying more attention to. Perhaps, like many groundbreaking ideas, it will slowly gain traction as physicists grapple with its implications over time.

Relating to Previous Discussions on Fundamental Physics
Readers following my previous articles on quantum physics such as “Loop Quantum Gravity vs. String Theory” or on advances in “Understanding String Theory”, might notice a through line: physicists globally continue to grapple with how to marry quantum mechanics with relativity. Wolfram’s theory raises many of the same questions addressed in string theory, yet ventures into completely new territory by attempting to use computational rules to explain everything. Similar to how loop quantum gravity discretizes space-time, Wolfram’s hypergraph approach seeks to unify fundamental physics but with a key computational twist.
The importance of finding a Theory of Everything cannot be overstated. It’s the holy grail of physics. Whether Wolfram’s computational universe is that missing link remains an open question, but it certainly warrants more attention as physicists and mathematicians further explore it.
Focus Keyphrase: Wolfram’s Theory of Everything