Tag Archive for: AI Predictive Models

Exploring Bayesian Probability in AI: A Gateway to Advanced Predictive Models

Deciphering the Intricacies of Bayesian Probability in Artificial Intelligence

In the realm of Artificial Intelligence (AI) and Machine Learning (ML), understanding the nuances of mathematical concepts is paramount for driving innovation and solving complex problems. One such concept, grounded in the discipline of probability theory, is Bayesian Probability. This mathematical framework not only offers a robust approach for making predictions but also enhances the decision-making capabilities of AI systems.

The Mathematical Framework of Bayesian Probability

Bayesian probability is a subfield of probability theory which interprets probability as a measure of belief or certainty rather than a fixed frequency. This perspective allows for updating beliefs in light of new evidence, making it an immensely powerful tool for prediction and inference in AI. The mathematical backbone of the Bayesian approach is encapsulated in Bayes’ Theorem:

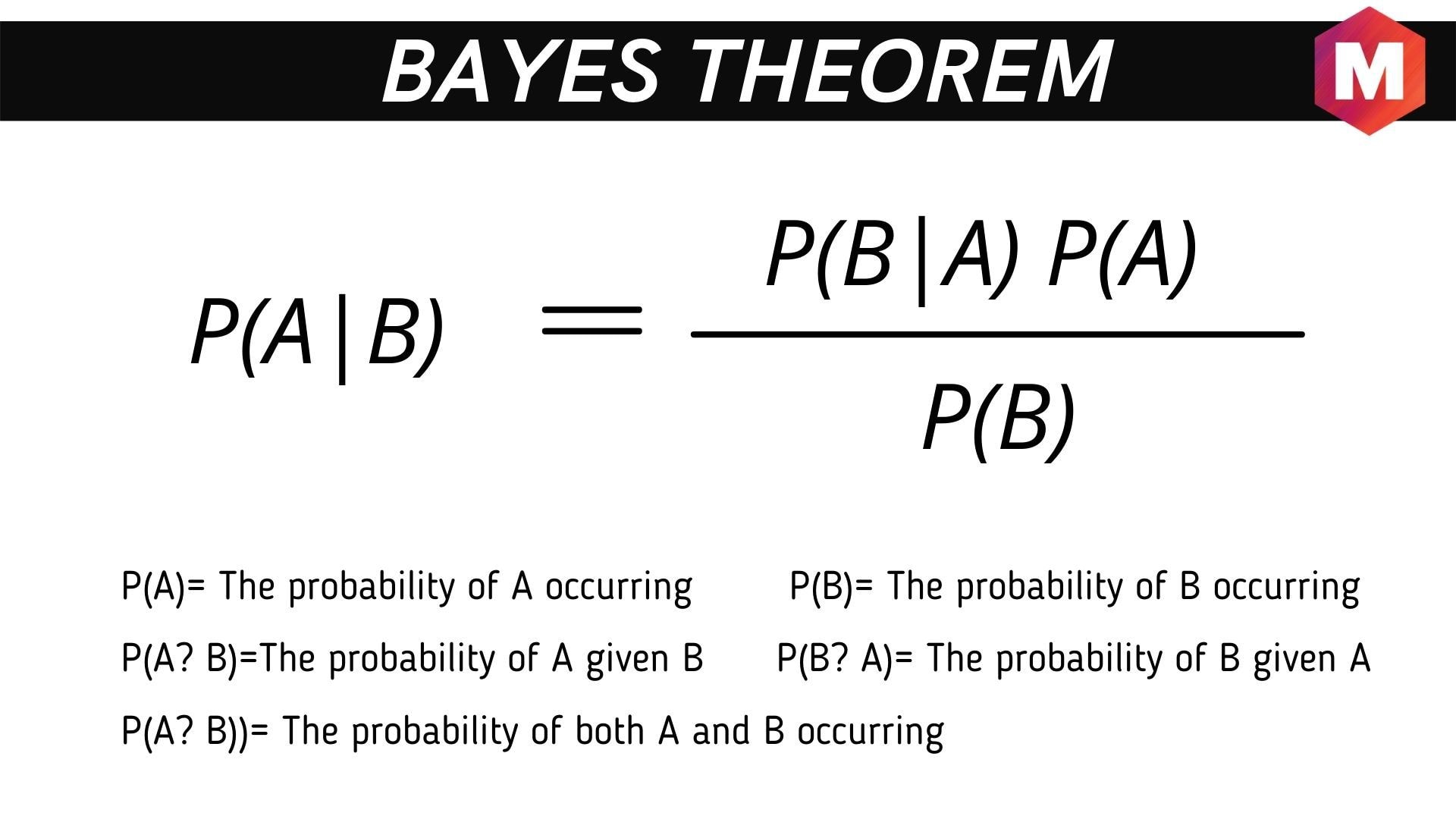
Bayes' Theorem Formula

In mathematical terms, Bayes’ theorem can be expressed as:

P(A|B) = (P(B|A) * P(A)) / P(B)

Where:

  • P(A|B) is the posterior probability: the probability of hypothesis A being true given that B is true.
  • P(B|A) is the likelihood: the probability of observing B given hypothesis A is true.
  • P(A) is the prior probability: the initial probability of hypothesis A being true.
  • P(B) is the marginal probability: the total probability of observing B.

Application in Artificial Intelligence

My work at DBGM Consulting, Inc., particularly in AI workshops and the development of machine learning models, heavily relies on the principles of Bayesian probability. A hallmark example is its application in predictive machines, such as chatbots and self-driving robots, which my team and I have developed using Bayesian frameworks for enhanced decision-making capabilities.

Consider a chatbot designed to provide customer support. Utilizing Bayesian probability, it can update its responses based on the interaction history with the customer, thereby personalizing the conversation and increasing the accuracy of its support.

Furthermore, Bayesian probability plays a crucial role in the development of self-driving robots. By continuously updating the robot’s knowledge base with incoming sensor data, we can predict potential hazards and navigate effectively—an application witnessed in my AI-focused projects at Harvard University.

Probability Theory in the Context of Previous Articles

Relating the principles of Bayesian Probability to my earlier discussions on the mathematical foundations of large language models, it’s evident that probability theory is paramount across the spectrum of AI research and development. Similar to how prime factorization in number theory secures cloud technologies, Bayesian inference ensures the AI’s decisions are both rational and data-driven.

Conclusion

Beyond its application in AI, Bayesian probability reminds us of the power of adaptability and learning from new experiences—a principle I embody in both my professional and personal pursuits. Whether it’s in crafting AI solutions at DBGM Consulting or delving into the mysteries of the cosmos with my amateur astronomer friends, the Bayesian approach provides a mathematical foundation for evolving our understanding with every new piece of evidence.

As we continue to explore the intricate dance between AI and mathematics, it becomes increasingly clear that the future of technological innovation lies in our ability to intertwine complex mathematical theories with practical AI applications. Bayesian probability is but a single thread in this vast tapestry, yet it’s one that weaves through many of the advances we see today in AI and beyond.

Focus Keyphrase: Bayesian Probability in AI

/2 Comments/by

Harnessing Numerical Methods for Superior AI Predictive Models

“`html

The Elegance of Numerical Methods in AI Predictive Models

In the realm of Artificial Intelligence and Machine Learning, precision and accuracy are paramount. It’s here that numerical analysis not only finds its application but also shines, bridging the gap between theoretical models and real-world data. As a consultant specializing in AI, I’ve witnessed firsthand the transformative power of numerical methods in predictive modeling.

Understanding Numerical Analysis

Numerical analysis is a branch of mathematics that devises, analyzes, and implements algorithms for obtaining numerical solutions to problems inherent in continuous mathematics. This discipline is the backbone of computational models, especially in areas requiring the solution of complex equations that are difficult or impossible to solve analytically.

Case Study: Solving Differential Equations

One of the most compelling examples of numerical analysis in action is in solving differential equations for predictive models. Differential equations are vital in modeling real-world processes in physics, engineering, and notably, in AI predictive models. The Role of Differential Equations in Enhancing AI Predictive Models further elucidates their importance.

Numerical Methods for Differential Equations

There are several numerical methods available for solving differential equations, such as Euler’s method, the Runge-Kutta methods, and the finite element method. Each has its advantages and specific use cases, but all serve the purpose of approximating solutions to otherwise intractable models.

Euler's method visualization

Let’s delve a bit deeper into Euler’s method, a fundamental numerical approach for solving ordinary differential equations (ODEs). It proceeds as follows:

  • Given an initial value problem \(y’=f(t,y), y(t_0)=y_0\),
  • Euler’s method approximates y at subsequent points by \(y_{n+1} = y_n + h*f(t_n, y_n)\),
  • where \(h\) is the step size and \(t_{n+1} = t_n + h\).

Although simple, Euler’s method lays the foundation for more complex algorithms and illustrates the fundamental idea of progressing step-wise to approximate solutions over a range.

Applying Numerical Methods in AI

My experience at DBGM Consulting, Inc., particularly in developing machine learning models for self-driving robots, highlighted the indispensability of numerical methods. For instance, optimizing pathfinding algorithms or sensor data interpretations invariably resorts to solving differential equations, for which numerical analysis provides the tools.

Pathfinding algorithm visualization

Numerical analysis, especially in AI, isn’t just about finding solutions—it’s also about ensuring these solutions are computationally feasible and aligned with real-world data. In essence, numerical methods help translate mathematical models into actionable intelligence, crucial for anything from process automation to predictive modeling.

Conclusion

The fusion of numerical analysis with AI and machine learning brings forth solutions that are both innovative and grounded in mathematical rigor. As someone who has navigated the intersection of technology, mathematics, and real-world application, I assert the value of numerical methods is immeasurable. They empower us to solve complex problems, optimize algorithms, and ultimately, drive forward the frontier of what’s possible in AI and beyond.

Machine learning model visualization


“`

/0 Comments/by
Link to: Get in touch

Let’s collaborate!

Contact Me

DAVID MAIOLO

 The content on this website, including text, photographs, and any other media, is the property of David Maiolo unless otherwise noted. No part of this website may be reproduced, distributed, or transmitted in any form or by any means, including photocopying, recording, or other electronic or mechanical methods, without the prior written permission of the owner.

DISCLAIMER

The information provided on this website is for general informational purposes only. While I strive to keep the information up-to-date and correct, I make no representations or warranties of any kind, express or implied, about the completeness, accuracy, reliability, suitability, or availability with respect to the website or the information, products, services, or related graphics contained on the website for any purpose. Any reliance you place on such information is therefore strictly at your own risk. In no event will I be liable for any loss or damage including without limitation, indirect or consequential loss or damage, or any loss or damage whatsoever arising from loss of data or profits arising out of, or in connection with, the use of this website. *This website may include links to other websites which are not under the control of David Maiolo. I have no control over the nature, content, and availability of those sites.

© 2024 David Maiolo